Product Maturity, Volume 2

3Non-Regression Tests

This chapter aims to verify whether the new definitions of products, subsets or components are at least as good as the previous ones. As already noted, two cases are possible.

3.1. Non-regression on a physical quantity

The assumption made here is that the two definitions can be modeled by normal distribution. This point must be reinforced by a test of normality. To compare two samples of observations, the classical approach involves using a central tendency measure, which is generally the mean. Therefore, a comparison of two means will be made.

The two underlying hypotheses are:

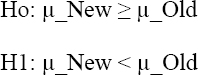
To illustrate the proposed method, let us consider the data in Table 3.1.

First, a test of normality should be conducted on the two variables (see Figures 3.2 and 3.3).

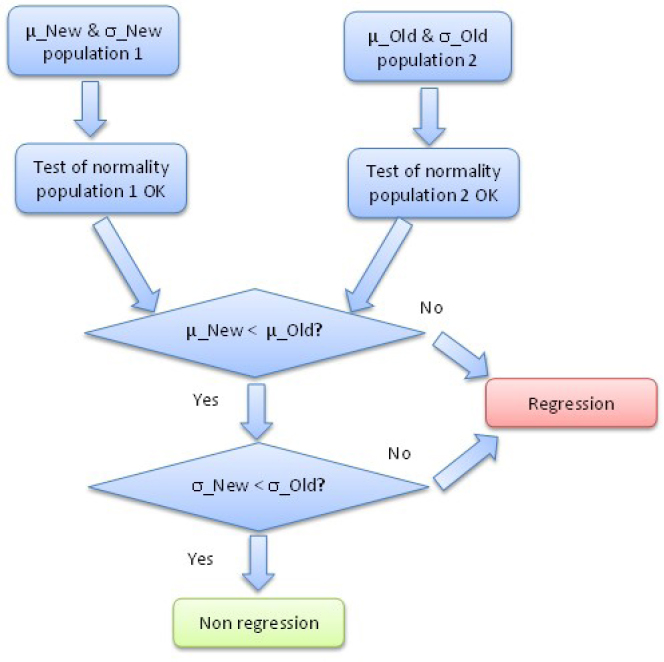
Figure 3.1. Overview diagram of non-regression on a physical quantity

Table 3.1. Example of a non-regression test

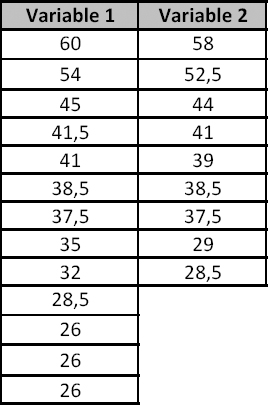
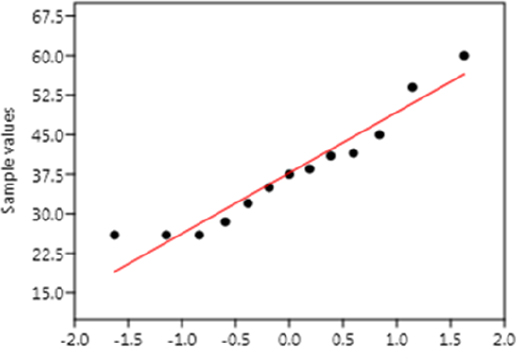
Figure 3.2. Test of normality on variable 1

Figure 3.3. Test of normality on variable 2

The ...